|
[HomePage] |
Professor W.W. Sawyer | |
Things and Unthings - An approach to negative numbers
There are several well-known ways of explaining negative numbers - earnings and debts, east and west. These work
quite easily for addition and subtraction but are rather troublesome for multiplication and division.
Now, of course, since in this world we cannot have -3 apples, there must be some sense of unreality connected with
negative numbers. I am about to describe a universe in which you could have -3 apples. This is a little fantastic
but that, if anything should commend it to children. But once we have qot this universe imagined, there is no cheating.
In it, children could learn to add and multiply negative numbers with the same apparatus and procedures that actual
children use in Grade 1 for actual numbers.
Grade 1 arithmetic is really a series of experiments in physics. You put two apples and two apples together and
find you have four apples. Or, starting with four apples you can break them up into two apples and two apples.
I will write in the form 2 T & 2 T <---> 4 T the fact of physics that two things and two things can be
combined into four things and vice versa. Children are thus led to write the arithmetical statement 2+2 = 4.
We now make our break with the accepted physics, and suppose the world contains not only things but also unthings.
If an un-thing and a thing meet, they wipe each other out. An un-apple wipes out an apple and an un-dog wipes out
a dog, and so on. It can work the other way round too. If you have a box with nothing in it, a little later you
may find that it contains a tiger and an un-tiger or a dollar and an un-dollar. (This latter fact raises special
problems in connection with forgery.)
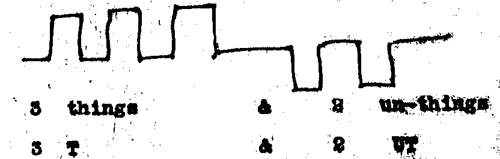
The illustration above shows 3 things and 3 un-things. An un-thing is written UT for short. 3 things and two un-things
can change, reversibly, into 2 things and 1 un-thing, or simply into 1 thing. In symbols
3 T & 2 UT <---> 2 T & 1 UT <---> 1 T
Use of the symbol U.
If X stands for some collection of objects, UX stands for what would wipe that collection out. Thus it D stands
for dog, and C for cat, U (C & D) stands for what would wipe out a cat and a dog. Clearly, that is an un-cat
and an un-dog, i.e.
UC & UD. so U(C & D) = UC & UD. U obeys the distributive law.
Now, what about U ( UC )? This means something that wipes out an un-cat. But that is a cat; for when a cat and an un-cat meet, they combine to form emptiness.
So U (UC) = C.
Un-Seven.
Th answer to, a question, "How many?". is a number. Suppose we are asked, "How many cats in this
room?" and there are in fact seven un-cats in the room. I suggest that the answer should be "Un-seven".
We may write this number u7. So "seven un-cats" and
"un-seven cats" are simply two ways of saying the same thing.
7 UC = u7 C
Adding and Subtracting.
Adding and subtracting can now be done in the usual way. For example, what is
3 + u5? Get 3 cats and 5 un-cats. Three of the un-cats will
wipe out the three cats, and in no time you be left with two un-cats.
3 C & 5 UC <---> 2 UC
3 + u5 = u2
.
Answer; un-two.
For subtraction; what is 3 - u5? You have 3 cats and you you want to give someone 5 un-cats; what will you have left afterwards? Well, take your 3 cats and stir them up a bit ; with luck they will change into 8 cats and 5 un-cats. Give away the 5 un-cats. You are left with 8 cats.
Answer, eight.
Alternative method, ask, "Un-five cats and what makes three cats?"
Multiplication.
This too is done in the usual way. It we want to, find what 3 x 2 is, we first take two things, TT; then we take
three sets of two things, TT TT TT. This gives us six things.
As 3 . (2 T) <---> 6T, we say 3 x 2 = 6.
Exactly the same method will work it we want (u3)
x (u2).
We must form un-three sets of un-two things each. Un-two things is the same as two un-things (see above, in paragraph
headed "Un-seven". ).
So un-two things is the set UT & UT. We want un-three such sets.
This is the same as three un-sets The un-set is T & T, or 2 T.
Three of these gives 6 T - Thus (u3) . (u2 T) = 6 T and we say (u3) x (u2) = 6 .
One could also carry through this argument by using the kind of diagram shown earlier, with things as mountains
and un-things as valleys into the mountains may fall. This would be probably easier as follows.
3 x (u2) and (u3) x 2 can be done in the same way.
It only remains to identify u3 with the usual sign -3.
Since 3 things and un-three things combine to give emptiness, it is clear that
3 + (u3) = 0. Thus u3 = 0 - 3; u3 is the result of subtracting 3 from zero.
This is ordinarily called -3.
The use of u allowed us to distinguish between un-three (negative 3; -3) and "subtract 3".
Un-three cats is an object. "Take away three cats" is an order.
Un-Things and Electrons.
As a concluding note it may be be pointed out that un-things are perhaps not so fantastic after all. In Dirac's theory of electrons, there were things called "electron-holes" which, when they met electrons destroyed the electrons and themselves ceased to be. Of course, this annihilation of matter produced considerable radiation. If one ignored the radiation effects, one would have an actual physical model for things and un-things.
Copyright © W. W. Sawyer & Mark Alder 2001
This version 23th February 2001
|